Every so often, someone besides myself will chime in on the potential futility of URR analysis for estimating peak oil dates. I tend to side with these critics, but not for the reason they have in mind. As a case in point consider this recent post from the
PealOil.com message board.
I see. Well, with that in mind, let me volunteer an interesting little article I recently saw in some volume or another of Natural resources Research or some such technical rag. Maybe any of the other people who actually read the research on this topic can chime in and correct me if I paraphrase it incorrectly. This guy fit all sorts of the Hubbert curve stuff to oil production, the standard curve fitter type stuff which have been used to depict the end of world, over and over again, except he measured the goodness of fit of ANY of these curves when using different ultimates. He used Hubberts ultimate, USGS ultimate, any other ultimate anyone had recently suggested, and he discovered that the same curve which fit one estimate of ultimate could fit darn near any others, even with these huge, and some would claim, meaningful differences. Apparently, these ultimate differences didn't matter much at all...literally. If you would enjoy a good read which quite reasonably dissembles the curve fitting approach, I'll go look it up as a reference for you.
I highlighted a portion that I agree with -- but for all the wrong reasons.
The commenter asserts that widely different parameterized curves, using a single parameter such as URR, can fit the same data equally well. Fair enough. But consider the case where we have a production curve that drops off as
1/t where
t=Time. If we follow the progression of this curve it monotonically decreases each year -- in other words, year after year
ad nauseum. Unfortunately, the ultimate cumulative (the URR) for this curve happens to equal infinity! Try as you might, you cannot argue this result, as this property comes as a mathematical given. Take a look at the curve below -- a simple hyperbola -- and you would perhaps imagine that the area under the curve, the cumulative production, had a finite value. Nice try, but wrong.

From this simple thought experiment, I find it not at all difficult to comprehend that a number of curves with vastly different URR's will fit the data equally well. After all, the difference between a finite URR and an infinite URR remains infinite, or at least some large number depending on how fast production falls off! Which is exactly the depletion dynamics the commenter complains about, give or take the unnamed article he refers to.
However, this should not obscure the fact that production indeed decreases each year after we have hit the peak for the
1/t curve. So whether or not we have an infinite URR does not matter when we have a decreasing yearly supply in the face of a yearly increasing demand for oil.
Which brings us to the real issue. I think that many oil depletion analysts over-rely on the URR approach and risk missing the forest for the trees. The usual heuristic applied, that the
peak occurs when cumulative production has hit URR/2, will not work in many cases, and will actually likely fail in every case of an asymmetric production curve. So for a constant URR, if we do indeed have longer tails then the peak occurs at < URR/2 (i.e. earlier), while if the tail shortens up then peak occurs > URR/2 (i.e. later). In the former case, it becomes a case of a terminal foreboding, while in the latter you have optimism right until you get hit by a truck traveling 80 MPH.
If that doesn't strike you as pedantically convincing, I would suggest starting with the
oil shock model, which does not use the questionable empiricism of the URR heuristic and work the model forwards. Becauses it instead uses historical discovery data, the impact of declining production rates doesn't get conflated with misguided assumptions related to the
ad hoc URR/2 peak value.
Let's get smart about this.
 I believe the 'minionists have finally reached a critical mass; as to what that entails, I don't think they even fathom. Neither do the nimrods at Powerline. Ha Ha.
I believe the 'minionists have finally reached a critical mass; as to what that entails, I don't think they even fathom. Neither do the nimrods at Powerline. Ha Ha. 







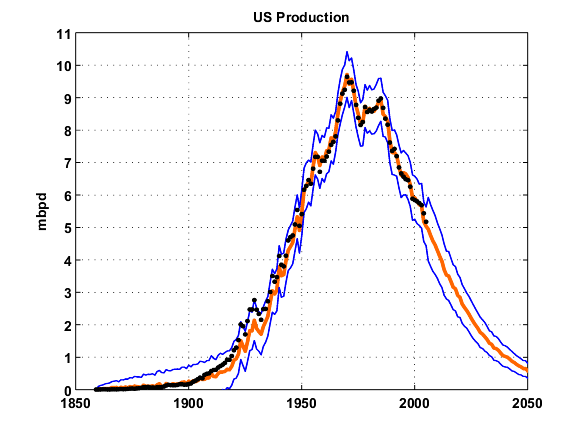
 But, hey, what the hay, I have a running blog to set things straight. So I redid the model with a "fallow" time constant of 1 year and a "construction" time constant of a smidge higher at 1.25. The resulting fit showed shocks much lower in extent, dropping from peak extraction rates of 0.3 to a more acceptable 0.08 per year value. As you can see, extraction peaked from the late 70's into the 80's and then dropped to a low right around the 1990 recession before rebounding during the 90's
But, hey, what the hay, I have a running blog to set things straight. So I redid the model with a "fallow" time constant of 1 year and a "construction" time constant of a smidge higher at 1.25. The resulting fit showed shocks much lower in extent, dropping from peak extraction rates of 0.3 to a more acceptable 0.08 per year value. As you can see, extraction peaked from the late 70's into the 80's and then dropped to a low right around the 1990 recession before rebounding during the 90's
 The "sniffing reporter" likely has support in the form of
The "sniffing reporter" likely has support in the form of  And another thing, anybody notice the striking resemblance between RNC chair Ken Mehlman (right) and the character named "Eb" (below) from
And another thing, anybody notice the striking resemblance between RNC chair Ken Mehlman (right) and the character named "Eb" (below) from 





 I find Mills' work the
I find Mills' work the